
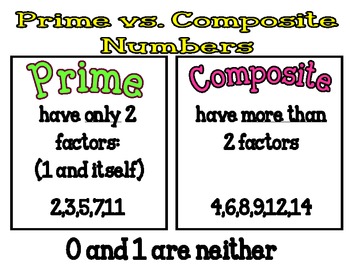
Is 0 a prime number? Is 1 a prime number? Let’s answer some common questions at this point. When you made your Sieve of Eratosthenes, did you notice that by finding the composite numbers, you also found the prime numbers? You’re likely to even start asking some of the same questions that have been catching the imaginations of mathematicians for thousands of years. Please? By going through the exercise, you’ll really get a deeper feeling for what prime numbers really are and how they work. It won’t take long, and you’ll learn a lot. We strongly recommend that you make a Sieve of Eratosthenes yourself. Way too much work!! Instead we can use an extremely clever technique called the Sieve of Eratosthenes. Using this logic, if we wanted to find all the composite numbers up to 100, we could make a 50 x 50 times table. In fact, we know that we’ve got all the composite numbers up through 20, because we’ve tried every number up through 10, and the smallest number they could be multiplied by was 2. However, we know that we do have all the composite numbers up to 10, because when you multiply numbers greater than 1 you always get numbers bigger than you started with. For example, 22 is a composite number (it’s 2 x 11), but it’s not in the table because we only multiplied numbers up to 10. Does The Table Show All The Composite Numbers up to 100? The table shows a whole bunch of composite numbers up to 100, but there’s a problem. All those numbers are composite numbers, because they are each products of two whole numbers greater than one. The table shows their products with each other in the violet colored middle of the table. The factors are the numbers with a white background on the top and left. We made it only using whole numbers greater than one. If you are interested in using a game to learn and practice, you can find it on the App Store. But, in order to truly be correct about it, we have to get into the details, and make sure to dot every i and cross every t.)īy the way, we created a math game to help students become good at breaking composite numbers into their prime factors, because it’s such an important skill. (The main idea is going to be that composite numbers have factors, and prime numbers don’t. If we weren’t worried about details like zero, one, fractions, and so forth, we could have just said: “When you multiply two numbers you get a composite number.” But, being that it’s math, we’re careful about pesky details, even at the expense of being a bit harder to understand. But, in mathematics, people try to make sure that they’re never wrong, even due to technicalities. In normal life, if you say something that’s pretty clear, and then a wise guy points out that you’re wrong due to a technicality, you just ignore the wise guy. That’s worth reading twice, because it’s a precise definition (again). Examples of Composite Numbers (As Shown by the Bubbly Primes Math Game)


 0 kommentar(er)
0 kommentar(er)
